C1.2 Create and translate patterns that have repeating elements, movements, or operations using various representations, including shapes, numbers, and tables of values.
Skill: Representing Patterns in Different Ways
Non-Numeric Patterns with Repeating Elements
Using multiple representations of the same pattern can support students in deepening their understanding. Non-numeric patterns with repeating elements can be represented using concrete or visual (semi-concrete) materials, symbols, and verbal descriptions.
Examples of Representation Modes
|
Representation Modes |
Examples |
|
Concrete Material |
Patterns made with: the body, such as sounds, movements or positions, or objects, such as necklaces Pattern A 
Pattern B 
|
|
Semi-Concrete Material |
Illustrated Pattern Pattern C 
|
|
Oral Description |
Pattern A: "It's a pattern because the standing arms outstretched and squatting positions always repeat in the same order." Pattern B: "The necklace makes a pattern because the four colours always repeat in the same way." Pattern C: "It's a pattern because the pattern core, which is a fire truck followed by a firefighter, always repeats in that order." |
|
Symbols |
Pattern A: The structure of the pattern is AB. Pattern B: The structure of the pattern is ABCD. Pattern C: The structure of the pattern is AB. |
To translate the representation of a pattern from one form to another involves maintaining the order of the repeating elements or the repeating operation from one position to the next.

Change can also occur within the same mode of representation.
Example


By representing a pattern with symbols, its structure can be clearly determined. To do this, letters are used in alphabetical order. Each new letter represents a different element in the pattern. In the patterns shown above, the structure is AABB.
Often, multiple representations of the same patterns are a good mathematical justification, as each contributes to the understanding of the ideas presented. The ability to create, interpret, and represent ideas in multiple ways is a powerful tool.
Source: translated from Guide d’enseignement efficace des mathématiques de la maternelle à la 3e année, Modélisation et algèbre, Fascicule 1, Régularités et relations, p. 34-36.
Pattern with Repeating Operation
Patterns with repeating operations can be represented using concrete or visual (semi-concrete) materials, symbols, or verbal descriptions.
|
Representation Modes |
Examples |
|
Concrete Material |
 |
|
Semi-Concrete Material |
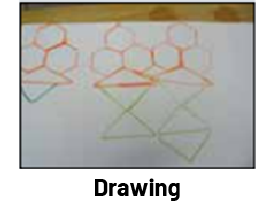 |
|
Oral Description |
"A trunk is always added to the trunk of the previous tree." |
|
Symbols |
In the drawing above, the repeat operation can be represented symbolically using the operation sign (e.g., addition signs) with its corresponding value (for example, + 1). |
In the sections which follow, we will deal more specifically with representations of non-numeric growing patterns using symbols and a table of values.
Symbols
Students use symbols to communicate the repeat operation. These symbols can be numbers as shown in Photo A with an understanding of the operation or can include the operation symbol such as the addition symbols (for example, +3) as in Photo B.
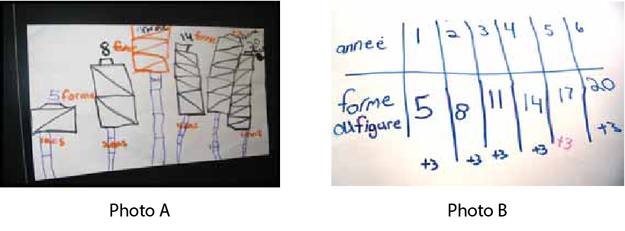
Table of Values
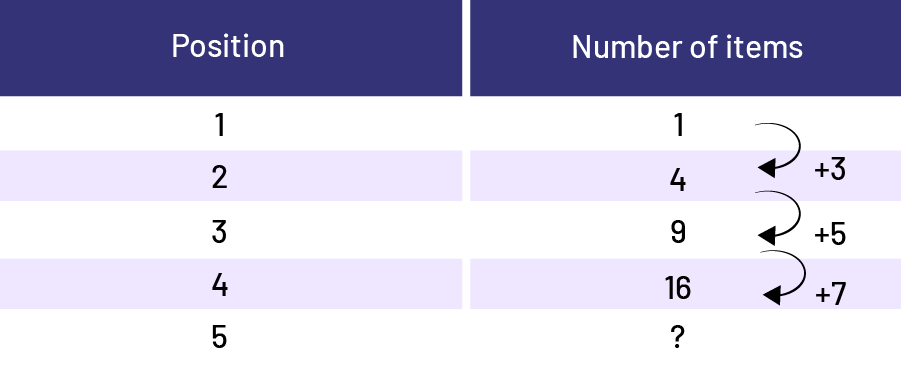
The table of values, introduced in Grade 3, is a numerical representation showing the value of each position. Translating from the picture to the table of values involves assigning each shape with a position (i.e., shape 1 at position 1, shape 2 at position 2…). The repeat operation can be determined by comparing the numerical values from one position to the next in the table of values.
Example

Horizontal Table of Values
| Number of years | 1 | 2 | 3 | 4 | 5 | 6 |
|---|---|---|---|---|---|---|
| Number of shapes | 5 | 6 | 7 | 8 | 9 | 10 |
| Number of years | Number of shapes |
|---|---|
| 1 | 5 |
| 2 | 6 |
| 3 | 7 |
| 4 | 8 |
| 5 | 9 |
| 6 | 10 |
The table of values can be arranged vertically or horizontally and separated into columns or rows. It is a good idea to vary the layout of the table of values so that students get used to both layouts.
In searching for patterns, some students will use concrete or semi-concrete material, others the table of values. It is important that students realize that the patterns found exist in both modes of representation.
Teachers should provide opportunities for students to explore and create different representations of the same pattern. For example, they may be asked to exchange their table of values with a peer and translate the representation of the pattern into concrete or semi-concrete form, and vice versa. It is important to focus on the connections between the two representations so that the emphasis is on reasoning and not on numerical calculation.
In Grade 3 , students use a table of values to represent a pattern with repeating operations in order to extend or determine the unknown value of a certain position number. It is helpful to model the use of tables of values so that students understand how to read and compose one.
Examples
- Concrete representation of a non-numeric pattern using pattern blocks.

- Representation of the same pattern using a table of values.
Source: translated from Guide d’enseignement efficace des mathématiques de la maternelle à la 3e année, Modélisation et algèbre, Fascicule 1, Régularités et relations, p. 41-44.
Number Patterns
The acquisition of concepts related to numeric patterns is a prerequisite to the study of more abstract algebraic concepts. The exploration of various representations of number patterns facilitates the entry into the world of algebra.
In the primary grades, certain representations facilitate the development of problem-solving, reasoning and communication skills. The representations of number patterns explored in the Primary Division are made using concrete or semi-concrete materials (table of values, number line, number grid), symbols and oral descriptions.
Examples of Representation Modes
|
Modes of representation |
Examples |
|
Concrete Material |
   |
|
Semi-Concrete Material |
|
|
Oral Description |
"The number pattern is: 6 vegetables the 1st week, 12 vegetables the 2nd week, 18 the 3rd week, 24 the 4th week and 30 vegetables the 5th week. The rule my vegetable garden presents is always 6 more vegetables than the previous week." |
|
Symbols |
In the previous example, the pattern rule in the number pattern is represented by the sign + and the number 6, that is +6. |
Representations of number patterns are made using a variety of concrete and semi-concrete materials. In the following, the types of manipulatives listed below will be used to further the understanding of number patterns:
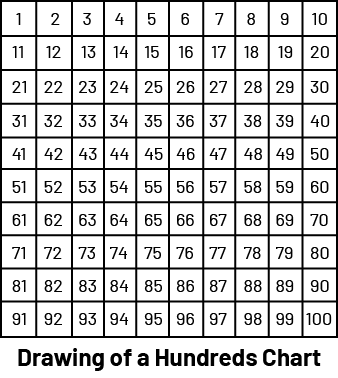
- hundreds chart;
- number grid (e.g, hundred chart);
- number line;
- a table of values
- calculator.
Hundreds Charts
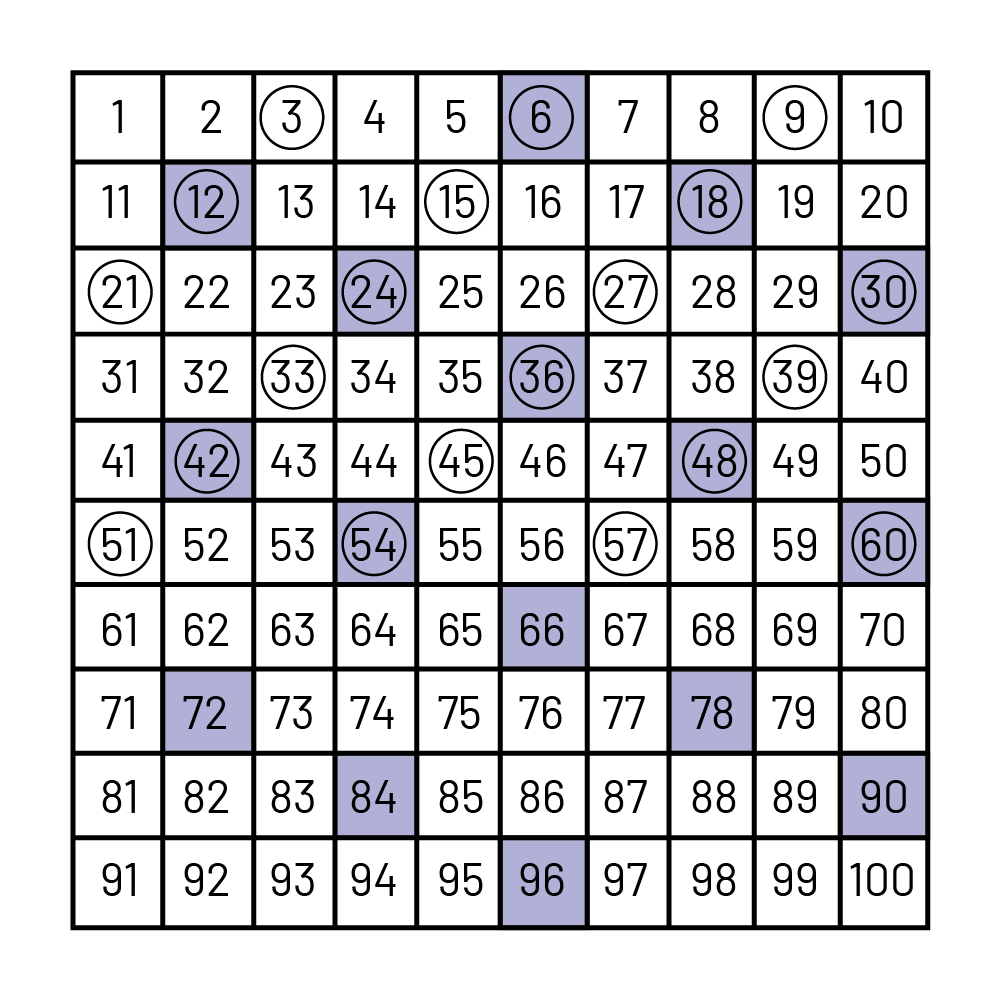
There are many patterns in a hundreds chart, and many arrangements of the patterns; for example, in a hundreds chart such as the one shown below, a pattern with the + 2 pattern rule will be arranged in a position (61, 63, 65…); if the pattern rule is + 10, the pattern will be arranged in a column (7, 17, 27…); if the pattern rule is + 11, it will be arranged in a slanting line (1, 12, 23…). In a number hundreds chart, number cards can easily be moved, rotated, or removed to create patterns.

Number Grid
Here are some possible strategies for exploring number patterns with a hundreds chart:
- Use bingo chips to record a pattern on a hundreds chart. Using tokens allows students to make changes without having to erase.
Example 1
- Use a masking card and move it around the grid to highlight a number pattern. Determine the pattern rule in this pattern and extend it.
Example 2
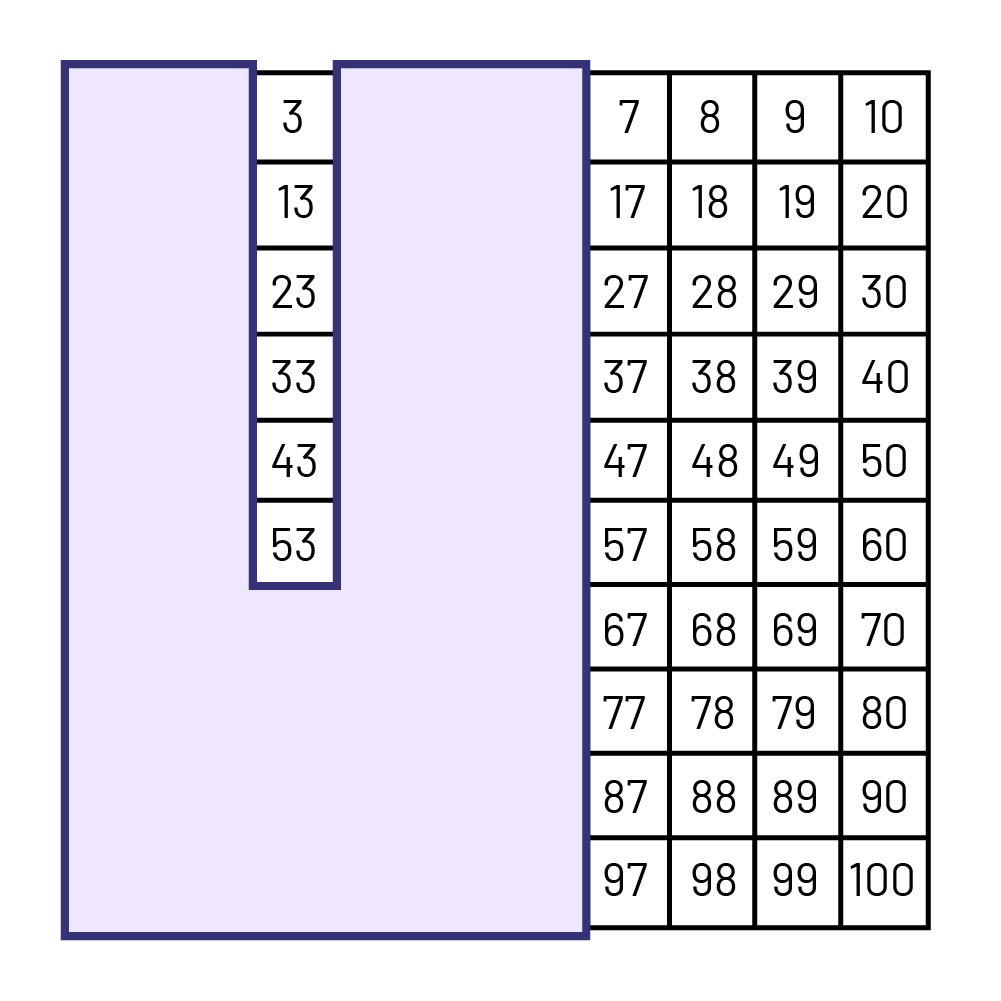
Pattern: 3, 13, 23, 33, 43, 53, 63, 73, 83, 93
Pattern Rule: +10
- Introduce sections of the grid and identify a number pattern. Point out the pattern rule in this pattern.
Example 3

Pattern: 18, 27, 36, 45
Pattern Rule: +9
When exploring number patterns on a hundreds chart, ask questions to develop the ability to represent them. For example:
- What do you notice about the numbers in the following?
- What change is there from one number to another in each position or column? (They increase or decrease by 1 or 10.)
- If the numbers were listed only in the first three rows, how would you determine which box contains the number 65?
- Where would the number 105 be if the chart was extended? How do you know?
- If the masking card is moved and the first number is 5, will the number 46 appear? Explain your answer.
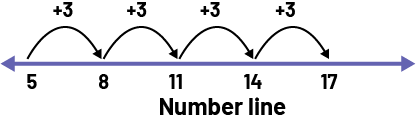
The Number Line

Used as a model for discovering pattern rules in patterns, the number line helps to represent a variety of patterns. The representation of the number line can be concrete or semi-concrete.
Here are some possible strategies for exploring number patterns with the number line:
Initially, use a laminated number line on which students can jump on the numbers at regular intervals, either by jumping or with some object.
Using a number line drawn on a large sheet of paper, students circle the terms in the pattern and indicate the rule in the pattern with an arrow.
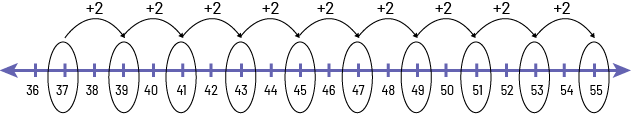
As students explore number patterns using a number line, ask questions such as:
- Would the number 58 be part of the pattern if we extended the line?
- What do you notice about the circled numbers on the number line?
- What is the pattern rule in the following? How is it indicated?
- If the pattern began with the number 1, what would be the same? different?
- If the pattern began with the number 2, what would be the same? different?
Table of Values
It is a model that establishes a relationship between a concrete representation and an abstract representation. In the junior grades, it will provide access to a graphical representation and allow students to study pattern rules. By examining the relationship between the position and the term, junior students will determine the functional pattern rule.
When students create a pattern and convert it to a number pattern in a table of values, it is easier for them to analyze the relationships and justify them.
Example


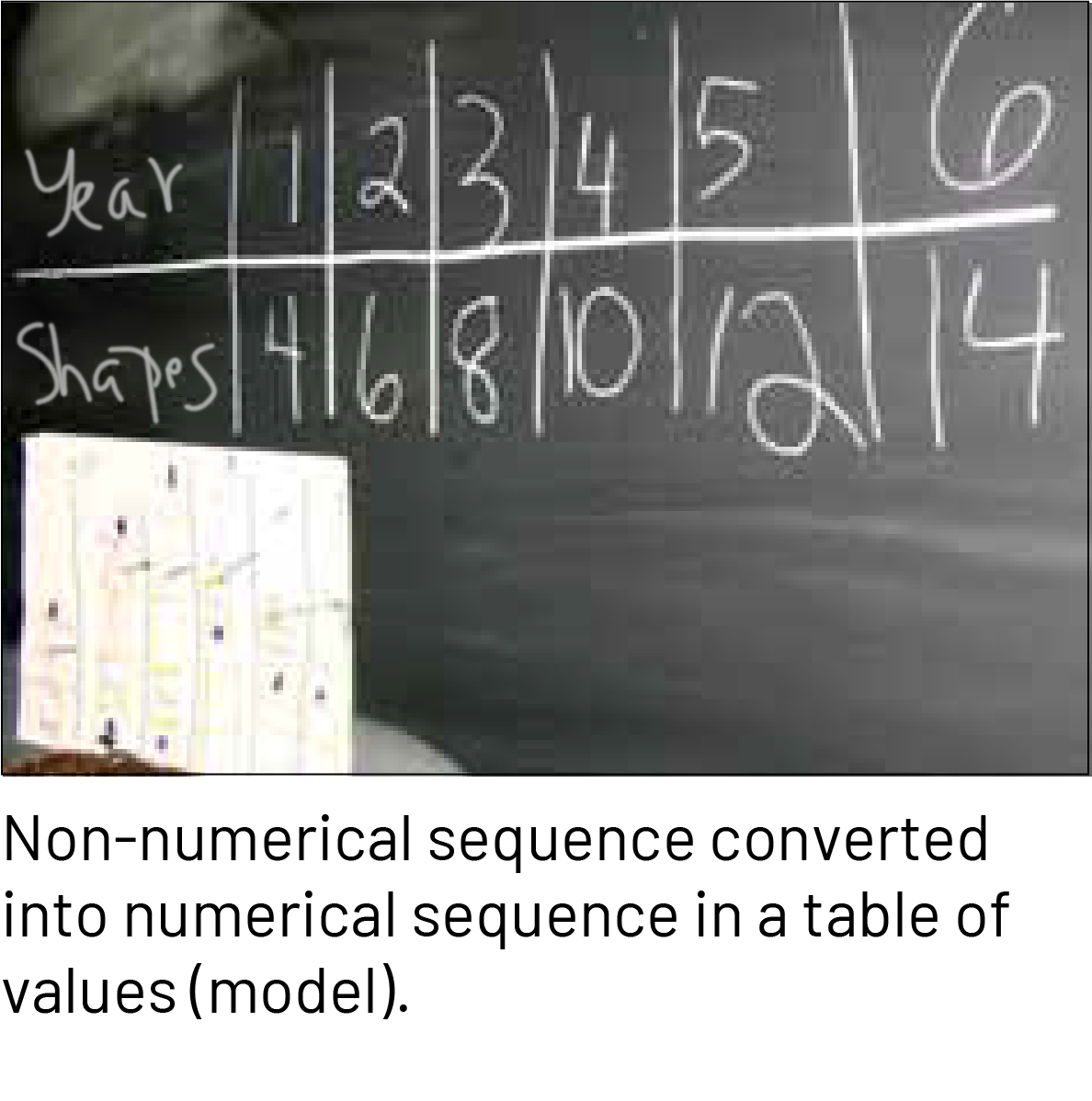
Ask questions to get students to justify the meaning of the numbers in the table of values. For example:
- What do the numbers in the 2nd position of the table of values represent?
- Can you show me on the concrete representation of what the number 7 represents in the table of values?
- What change do you notice from one position to the next?
- Why is it important to indicate the age of the tree in the table of values?
- What would be the corresponding number in the 2nd position?
- What do the words shapes and tree age represent?
- What can we say about the pattern of numbers in the table of values?
- Can you explain the reason for listing four shapes under the number 1?
Calculator
This electronic tool allows you to explore number patterns and easily extend patterns using the Constant Factor function. It is important to model the data input with a virtual calculator beforehand.
Example 1
Choose a starting number between 0 and 9. Then add a number to that number continuously. For example:
- Press the numeric key [7].
- Add an interval of 4 by pressing the [+] key, then the numeric key [4].
- Press the [=] key repeatedly.
- Write the corresponding number pattern on a sheet of paper as you go along (in this case: 7, 11, 15, 19, 23…).
- Indicate the pattern rule in the following (+4).
Ask thought-provoking questions such as:
- Which numbers always appear in the units position? What changes when you change the starting number or the selected interval?
- How many terms does the pattern contain before a unit digit repeats?
- Do you think that if you count by 4, the number 37 will appear? How do you know that?
Example 2
Create, as in example 1, a pattern whose starting number is 23 and whose pattern rule is +12 (23, 35, 47, 59, 71…).
Ask questions such as:
- Is there a pattern rule in this pattern? What is it?
- If the pattern was extended indefinitely, would the number 155 be part of the pattern? What do you have to do to find out?
Example 3
Create, as in the previous examples, a pattern whose starting number is 11 and whose pattern rule is +11 (11, 22, 33, 44, 55…).
Ask questions such as:
- If we extend the pattern, what will be the term in the 7th position? The term in the 18th position?
- Will the number 222 be part of this pattern?
Source: translated from Guide d’enseignement efficace des mathématiques de la maternelle à la 3e année, Modélisation et algèbre, Fascicule 1, Régularités et relations, p. 51-59.
Skill: Creating Various Patterns
Before creating patterns, students must first recognize, compare, describe, translate the representation, and extend a wide variety of patterns.
Students demonstrate their understanding of the concept of pattern rule by creating a pattern and explaining it.
Initially, the use of manipulatives is essential for representing patterns, because when constructing patterns, students can easily change one element of the pattern and check the rule, whereas when drawing it on paper, they focus on the drawing to be reproduced rather than examining the entire pattern and thinking about the nature of the pattern rule.
Teachers have students work in small groups to encourage the exchange of ideas and can provide the attributes and structure of the pattern as well as the materials needed to create it.
During a mathematical exchange, presentations of the patterns make it possible to note the different representations of the same rule and to check the way in which the students communicate their understanding.
Next, students can be asked to create a pattern and exchange it with a peer. They can then construct a different representation of the received pattern, describe its structure, extend it, or produce a completely different one for comparison. The number of elements in the pattern should be limited, however, as some students use too many, making it difficult to understand the structure of the pattern.
Source: translated from Guide d’enseignement efficace des mathématiques de la maternelle à la 3e année, Modélisation et algèbre, Fascicule 1, Régularités et relations, p. 39-40.
Here are some examples of activities to create number patterns:
Example 1
Provide a pattern rule for addition or subtraction (for example, +3 or -3). Have students create different patterns with this pattern rule and compare them.
Example 2
On a hundreds chart, ask students to create two patterns in which the numbers 6, 12, 24, 42, and 54 are found and whose addition pattern rule is other than +1.

Note: The possible patterns which meet the two stated criteria are the patterns whose rule is +2, +3 or +6.
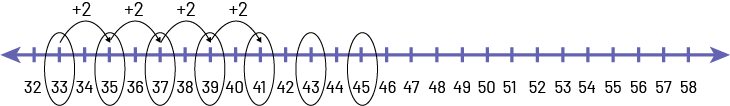
Example 3
Ask students to create two different number patterns in which the numbers 33 and 57 are found and whose addition pattern rule is other than +1.

Solution: The possible pattern rules for creating patterns that meet the stated criteria are +2, +3, +4, +6, +8, +12 and +24.
Note: It is important for students to leave a record of their process for reference during the mathematical exchange. For example, they can:
- circle the terms of each pattern to locate them;
- draw an arrow above each jump to indicate the interval;
- symbolically represent the pattern rule with a + sign, as in the example below.
Possible Solution
Source: translated from Guide d’enseignement efficace des mathématiques de la maternelle à la 3e année, Modélisation et algèbre, Fascicule 1, Régularités et relations, p. 63-64.
Skill: Making Connections Between Different Representations
When faced with a problem situation, several representations are possible; some students use their bodies, manipulatives or drawings, while others represent the data more schematically. The way in which data is appropriated and organized using models reflects the level of development of algebraic thinking. The models explored in the primary and junior grades will differ depending on the students' level of abstraction. The ten frame, the array, the table of values, the open number line, and the open double number line are models to be encouraged in the primary grades.
Teachers should use these models and introduce students to using them to help them reason. In representing a problem situation, students analyze relationships using models, draw conclusions, and explain them using oral descriptions. Models are tools that help students formalize their algebraic thinking.
Models applied to multiple contexts promote analysis and introduce students to a level of abstraction that facilitates predictions and generalizations. Dialogue, mathematical exchange about the problem data represented with different models, and questions from teachers provoke student reflection.
Source: translated from Guide d’enseignement efficace des mathématiques de la maternelle à la 3e année, Modélisation et algèbre, Fascicule 1, Régularités et relations, p. 18-21.
Comparing different representations of a pattern and between different patterns can provide a better understanding of their characteristics, promotes reflection, facilitates communication, and allows students to develop their algebraic reasoning.
Source: translated from Guide d’enseignement efficace des mathématiques de la maternelle à la 3e année, Modélisation et algèbre, Fascicule 1, Régularités et relations, p. 59.
Non-Numeric Repeating Patterns
During mathematical conversations, teachers should engage students in comparing the special characteristics of patterns such as:
- the attributes;
- the choice and quantity of elements in each pattern;
- the pattern core;
- the structure of each pattern.
Examples
|
Pattern 1 |
Pattern 2 |
 |
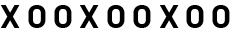 |
|
Attributes: Shape and Colour |
Attribute: Symbols |
|
Pattern core: one yellow circle then two blue trapezoids |
Pattern core: one x symbol then two o symbols |
|
Structure: ABB |
Structure: ABB |
Understanding the pattern core is essential to being able to extend and create patterns.
To get students to create a mental image of a pattern, teachers can also have students compare patterns using examples and non-examples of patterns. This strategy allows students to recognize a pattern, identify the pattern core, describe it and justify their reasoning while using appropriate mathematical vocabulary.
Example of a Pattern with a Repeating Core

Non-Example of a Pattern - No Pattern Core

Source: translated from Guide d’enseignement efficace des mathématiques de la maternelle à la 3e année, Modélisation et algèbre, Fascicule 1, Régularités et relations, p. 33-34.
Patterns with a Repeating Operation
The approaches described above for comparing repeating patterns also apply to patterns with a repeating operation.
Source: translated from Guide d’enseignement efficace des mathématiques de la maternelle à la 3e année, Modélisation et algèbre, Fascicule 1, Régularités et relations, p. 40.
Number Patterns
Comparing Two Patterns on a Number Grid
Comparing patterns on the same number grid allows us to establish relationships between numbers.
Example
Introduce students to the following two number patterns.
Pattern A: 3, 6, 9, 12, 15…
Pattern B: 6, 12, 18, 24, 30…
Use transparent tokens to represent pattern A and shade the appropriate boxes to represent pattern B in a hundreds chart.

Then, get students to think about and compare the patterns by asking questions such as:
- What do you observe once the two patterns are represented on the grid?
- Possible observations: some numbers are covered with a transparent token; some numbers covered with a transparent token are also shaded; the patterns form diagonal lines; there is always a difference of 3 between the numbers covered with a token; there is always a difference of 6 between the shaded numbers.
- Are there any numbers that are part of both patterns?
- What is the relationship between these numbers?
- What is the rule for each pattern?
- What similarity and difference is there between Pattern A: 3, 6, 9, 12, 15… and Pattern C: 5, 8, 11, 14, 17...?
Comparing Patterns On Different Size Number Grids
This allows us to deepen the concept of relationship between numbers.
Example
Present students with two number patterns written in grids of different widths like those shown below:
Pattern A: 4, 8, 12, 16… represented in Grid 1;
Pattern B: 3, 6, 9, 12… represented in Grid 2.
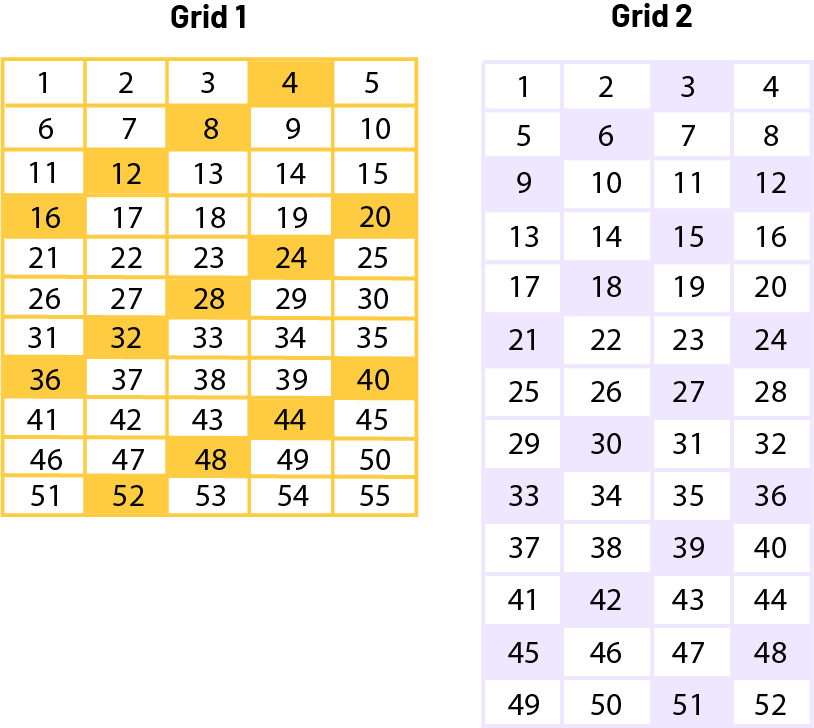
To provoke reflection, ask questions such as:
- What do you observe about the two patterns shown in the grids? (The patterns form diagonal lines; some numbers are overlapped in both grids.)
- What is the width of Grid 1? of Grid 2?
- What is the pattern rule for Pattern A? for Pattern B? (Pattern rule for pattern A: +4 or -4. Pattern Rule for pattern B: +3 or -3.)
Note: The subtraction pattern rule can be found if the pattern is read from bottom to top in the grid. For example, in Grid 1, the pattern can be read as follows: 52, 48, 44, 40, 36, 32, 28…
- Why are the two patterns that do not have the same pattern rule arranged in diagonal lines in the grids?
- If we change the width of Grid 1 (Grid 2), will the pattern still be laid out in a slanted right-hand direction?
- Which pattern rule would result in a pattern arranged in columns in Grid 1? in Grid 2?
- What would happen if we represented the same pattern in two grids of different widths?
Note: This activity is an exploration and observation of the relationships between numbers on grids of different widths. For example, the pattern 4, 8, 12, 16… placed on a grid with a width of 5 or a grid with a width of 9 will allow the observation of similar slanting lines.
Comparing Patterns Using Different Representations
Comparing different representations of the same pattern encourages the analysis of relationships.
Example
Pattern: 2, 4, 6, 8…
Represent this pattern on a hundreds chart and on a number line.
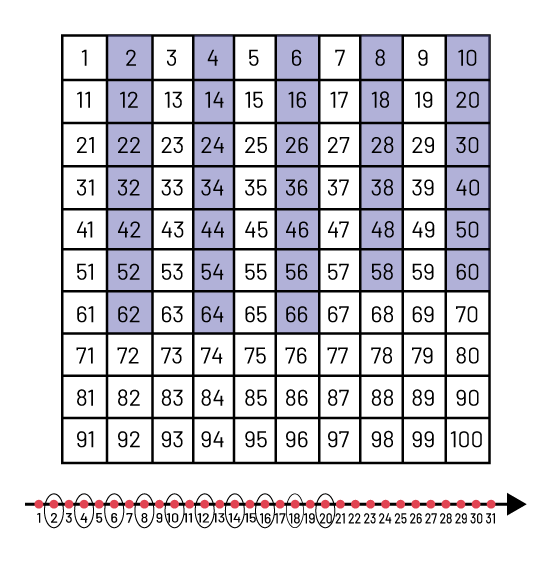
Make the connection between the two and compare the representations by asking questions such as:
- What change is there from one square to another on the grid? from one number to another on the number line?
- How many numbers are there between two consecutive shaded numbers on the hundreds chart?
- How many numbers are there between two consecutive circled numbers on the number line?
- What will be the next number shaded on the grid? circled on the number line?
- What pattern is represented?
- Which representation makes it easier to find the rule? Explain your answer.
- Can we always use a grid or a number line to represent a number pattern?
- If we wanted to represent more terms in the pattern on the number line, how should we modify it?
A calculator can also be used to represent the pattern.
Source: translated from Guide d’enseignement efficace des mathématiques de la maternelle à la 3e année, Modélisation et algèbre, Fascicule 1, Régularités et relations, p. 59-63.
Knowledge: Table of Values
The table of values is a numerical representation of a pattern. In the table of values, each shape is associated with a term (shape 1 at term 1, shape 2 at term 2…). This table makes it easier to identify number patterns and analyze change. After organizing the data in a table of values, students observe two representations of the same rule: the one created with materials or a drawing and the number pattern in the table of values.
The table of values can be vertical or horizontal.
| Number of cases | Number of lemons |
|---|---|
| 1 | 9 |
| 2 | 18 |
| 3 | 27 |
| 4 | 36 |
| 5 | 45 |
| 6 | 54 |
| 7 | 63 |
| Number of cases | 1 | 2 | 3 | 4 | 5 | 6 | 7 |
|---|---|---|---|---|---|---|---|
| Number of lemons | 9 | 18 | 27 | 36 | 45 | 54 | 63 |
Source: translated from Guide d’enseignement efficace des mathématiques de la maternelle à la 3e année, Modélisation et algèbre, Fascicule 1, Régularités et relations, p. 42-43.
